[math]\sqrt{x+3}>\sqrtx+\sqrt(2x-1)[/math]
[math]\sqrt{x+3}>\sqrtx+\sqrt(2x-1)[/math]
Per l'esistenza della disequazione deve essere:
[math]\begin{cases} x+3>=0 \\ 2x-1>=0 \\ x>=0 \ \end{cases}[/math]
;
[math]\begin{cases} x>=-3 \\ x>=1/2 \\ x>=0 \ \end{cases}[/math]
;
L'unione delle soluzioni sarà:
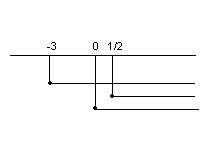
[math]S={x>=1/2}[/math]
In tale condizione i due membri sono positivi, elevando al quadrato si deve risolvere il sistema:
[math]\begin{cases} \sqrt{x+3})^2>(\sqrtx+\sqrt(2x-1))^2 \\ x>=1/2 \ \end{cases}[/math]
;
[math]\begin{cases} x+3>x+2x-1+2\sqrt{x(2x-1)} \\ x>=1/2 \ \end{cases}[/math]
;
[math]\begin{cases} \sqrt{x(2x-1)}>2-x \\ x>=1/2 \ \end{cases}[/math]
;
Eleviamo ancora al quadrato ambo membri della prima equazione:
[math]\begin{cases} x(2x-1)>(2-x)^2 \\ x>=1/2 \ \end{cases}[/math]
;
[math]\begin{cases} 2x^2-x>4-4x+x^2 \\ x>=1/2 \ \end{cases}[/math]
;
[math]\begin{cases} x^2+3x-4>0 \\ x>=1/2 \ \end{cases}[/math]
;
Studiamo la disequazione di secondo grado:
[math]x^2+3x-4>0[/math]
[math]Delta=b^2-4ac=3^2-(4 \cdot 1 \cdot (-4))=9+16=25[/math]
[math]x_(1,2)=(-b+-\sqrt{Delta})/(2a)=(-3+-\sqrt(25))/2=(-3+-5)/2 => x_1=-4 ^^ x_2=1[/math]
.
Siccome il segno del coefficiente di
[math]x^2[/math]
è concorde col segno della disequazione,
prenderemo gli intervalli esterni, quindi soluzione della disequazione sarà:
[math]x>-4 vv x>1[/math]
.
L'unione delle soluzioni, darà la soluzione finale
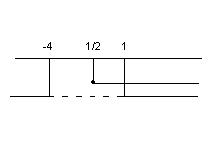
[math]S={x>1}[/math]
.
Accedi a tutti gli appunti
Tutor AI: studia meglio e in meno tempo
