[math]\sqrt{x^2-2x-1}>x-1[/math]
[math]\sqrt{x^2-2x-1}>x-1[/math]
;
L'indice della radice è pari ed è del tipo
[math]\sqrt{f(x)}>g(x)[/math]
quindi sarà equivalente al sistema:
[math]\begin{cases} f(x)>=0 \\ g(x)>0 \\ f(x)>[g(x)]^2 \ \end{cases}[/math]
;
Nel nostro caso
[math]f(x)=x^2-2x-1 ^^ g(x)=x-1[/math]
.
Quindi la disequazione è equivalente al sistema:
[math]\begin{cases} x^2-2x-1>=0 \\ x^2-2x-1>(x-1)^2 \\ x-1>0 \ \end{cases}[/math]
;
[math]\begin{cases} x^2-2x-1>=0 \\ x^2-2x-1>x^2-2x+1 \\ x>1 \ \end{cases}[/math]
[math]\begin{cases} x^2-2x-1>=0 \\ -1>+1 \\ x>1 \ \end{cases}[/math]
La seconda disequazione è verificata
[math]AA x in RR[/math]
.
Studiamo singolarmente la disequazione di secondo grado:
[math]x^2-2x-1>=0[/math]
[math](Delta)/4=(b/2)^2-ac=(-1)^2-(1 \cdot (-1))=1+1=2[/math]
[math]x_(1,2)=(-b/2+-\sqrt{(Delta)/4})/a=(1+-\sqrt2) => x_1=(1-\sqrt2) ^^ x_2=(1+\sqrt2)[/math]
.
Siccome il segno del coefficiente di
[math]x^2[/math]
è concorde col segno della disequazione,
prenderemo gli intervalli esterni, quindi soluzione della disequazione sarà:
[math]S_2={x>=(1-\sqrt2) vv x>={1+\sqrt2}}[/math]
.
Intersechiamo, ora, le soluzioni trovate e otterremo la soluzione finale del sistema
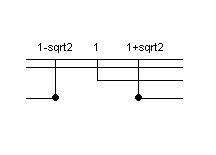
[math]S={x>=(1+\sqrt2)}[/math]
.
Accedi a tutti gli appunti
Tutor AI: studia meglio e in meno tempo
