di francesco.speciale

Ominide

4 min. di lettura

Vota 4,3 / 5

[math]|x-1|+2|x^2+5x+4|>6x^2-x[/math]

[math]|x-1|+2|x^2+4x+4|>6x^2-x[/math]

Studiamo il segno degli argomenti dei moduli
Primo modulo

[math]x-1>=0 => x>=1[/math]

Secondo modulo

[math]x^2+5x+4>=0[/math]

[math]Delta=b^2-4ac=(5)^2-(4 \cdot (4) \cdot 1)=25-16=9[/math]

[math]x_(1,2)=(-b+-\sqrt{Delta})/(2a)=(-5+-\sqrt9)/2=(-5+-3)/2 => x_1=-4 ^^ x_2=-1[/math]

Siccome il coefficiente di

[math]x^2[/math]

e il segno della disequazione sono concordi,
prenderemo come soluzione accettabile l'intervallo esterno,
per cui la soluzione sarà:

[math]x>=-4 vv x>=-1[/math]

.
Pertanto

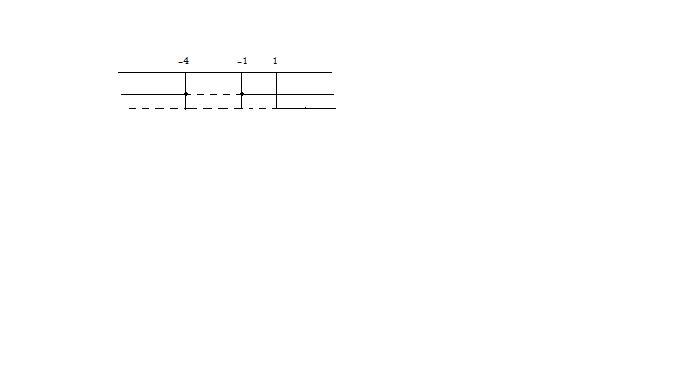
Dobbiamo distinguere quattro casi:
Se

[math]\begin{cases} x>=-4 \\ -x+1+2(x^2+4x+4)>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} x>=-4 \\ -x+1+2x^2+8x+8>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} x>=-4 \\ -4x^2+10x+9>0 \ \end{cases}[/math]

;

[math]\begin{cases} x>=-4 \\ 4x^2-10x-9>0 \ \end{cases}[/math]

;
Studiamo la disequazione di secondo grado:

[math](Delta)/4=(b/2)^2-ac=(-5)^2-((-9) \cdot 4)=25+36=61[/math]

[math]x_(1,2)=(-b/2+-\sqrt{(Delta)/4})/a=(5+-\sqrt(61))/4 => x_1=(5-\sqrt(61))/4 ^^ x_2=(5+\sqrt(61))/4[/math]

Siccome il segno del coefficiente di

[math]x^2[/math]

è concorde col segno della disequazione,
prenderemo gli intervalli esterni, quindi soluzione della disequazione sarà:

[math]x>(5-\sqrt{61})/4 vv x>(5+\sqrt{61})/4[/math]

.
Pertanto

[math]S_1=x>=-4[/math]

[math]\begin{cases} -4>=x>=-1 \\ -x+1-2(x^2+4x+4)>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} -4>=x>=-1 \\ -x+1-2x^2-8x-8>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} -4>=x>=-1 \\ -8x^2-10x-7>0 \ \end{cases}[/math]

;

[math]\begin{cases} -4>=x>=-1 \\ 8x^2+10x+7>0 \ \end{cases}[/math]

;
Come possiamo notare, la disequazione

[math]8x^2+10x+7>0[/math]

è verificata

[math]AA x in RR[/math]

.
Pertanto

[math]S_2=-4>=x>=-1[/math]

[math]\begin{cases} -1>=x>=1 \\ -x+1+2(x^2+4x+4)>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} -1>=x>=1 \\ -x+1+2x^2+8x+8>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} -1>=x>=1 \\ -4x^2+10x+9>0 \ \end{cases}[/math]

;

[math]\begin{cases} -1>=x>=1 \\ 4x^2-10x-9>0 \ \end{cases}[/math]

;
Quindi soluzione della disequazione sarà:

[math]x>(5-\sqrt{61})/4 vv x>(5+\sqrt{61})/4[/math]

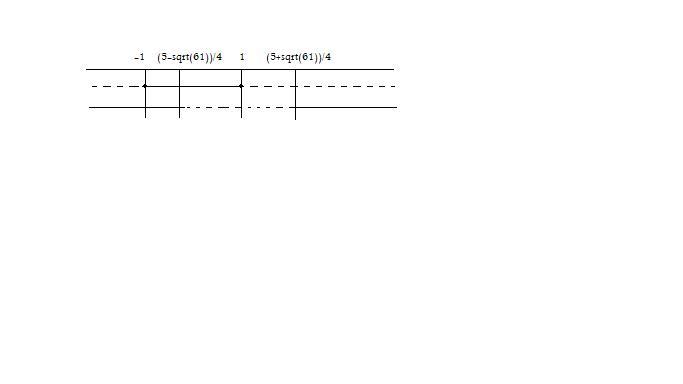
Pertanto

[math]S_3=-1>=x>(5-\sqrt{61})/4[/math]

[math]\begin{cases} x>=1 \\ x-1+2(x^2+4x+4)>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} x>=1 \\ x-1+2x^2+8x+8>6x^2-x \ \end{cases}[/math]

;

[math]\begin{cases} x>=1 \\ -4x^2+12x+7>0 \ \end{cases}[/math]

;

[math]\begin{cases} x>=1 \\ 4x^2-12x-7>0 \ \end{cases}[/math]

;
Studiamo la disequazione di secondo grado:

[math](Delta)/4=(b/2)^2-ac=(-6)^2-((-7) \cdot 4)=36+28=64[/math]

[math]x_(1,2)=(-b/2+-\sqrt{(Delta)/4})/a=(6+-\sqrt(64))/4=(6+-8)/4 => x_1=-1/2 ^^ x_2=7/2[/math]

Siccome il segno del coefficiente di

[math]x^2[/math]

è concorde col segno della disequazione,
prenderemo gli intervalli esterni, quindi soluzione della disequazione sarà:

[math]x>-1/2 vv x>7/2[/math]

.
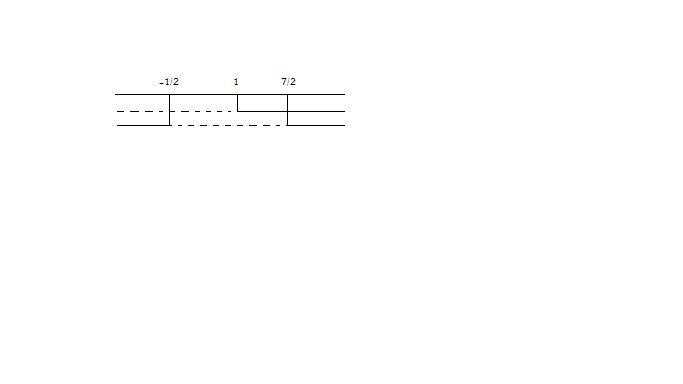
Pertanto

[math]S_1=x>7/2[/math]

Pertanto soluzione della disequazione iniziale sarà: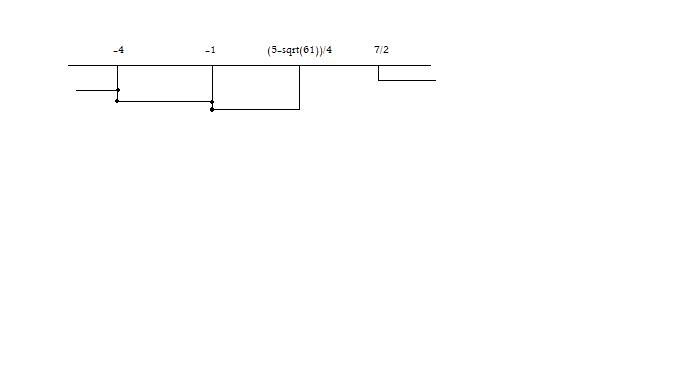

[math]S=x>(5-\sqrt{61})/4 vv x>7/2[/math]

Disequazioni: |x-1|+2|x^2+5x+4|

|x-1|+2|x^2+5x+4|<6x^2-x |x-1|+2|x^2+4x+4|<6x^2-x Studiamo il segno degli argomenti dei moduli Primo modulo x-1>=0 => x>=1 Secondo modulo x^2+5x+4>=0 Delta=b^2-4ac=(5)^2-(4(4)1)=25-16=9 x_(1,2)=(-b+-sqrt(Delta))/(2a)=(-5+-sqrt9

Recensioni

Domande e risposte

Help (321322)

Aiuto urgente per compiti

Problema con potenze

I migliori insegnanti di Matematica

Giovanni C.

Salvatore F.

Appunti correlati

Disequazioni: (x+1)^2+5x-1>(x-4)^2+2x-3

Disequazioni: (3x+1)^2-4x(x-2)>5x(x+6)

Disequazioni: 5x-6(x-1)>(x-3)(x+3)-(x-2)(x+2)

Disequazioni: {(x^2-5x+4>0),(x^2+5x+4 lt 0):}

Recensioni

Domande e risposte

Help (321322)

Aiuto urgente per compiti

Problema con potenze

I migliori insegnanti di Matematica

Giovanni C.

Salvatore F.